|
5.2. Localizations
An epi f: Y --> X is called essential
provided that for any map g: Z --> Y, if fg is epic
then g is epic.
Proposition 5.2.1. Suppose f:
Y --> X is a coflat map and X is integral.
(a} If f is an essential epi then Y is integral.
(b} If f is a bimorphism then Y is integral.
Proof. Let p: P(X) --> X be
the generic residue of the integral object X, which is an epi coflat
fraction by (5.1.2.c). Let (g:
Z --> Y, h: Z --> P(X)) be the pullback of (f:
Y --> X, p: P(X) --> X).
(a) Assume f is an essential epi. Since f and p
are coflat and epic, g and h are epic. Thus Z is non-initial,
which has a simple strong subobject q: Q --> Z (4.2.2.d).
Since P is simple, hq: Q --> P(X) is epic.
Since p is epic, phq = fgq is also epic. Since f is
an essential epi, gq: Q --> Y is epic. Then Y, as
a quotient of a simple object, is integral.
 (b) This follows from (a) as any coflat bimorphism is an essential epi.
by (1.4.4.e).
(b) This follows from (a) as any coflat bimorphism is an essential epi.
by (1.4.4.e).
Proposition 5.2.2. Suppose f:
Y --> X is any map. The image of Spec(f) is {V Î
Spec(X): f+1(f-1(V))
= V}.
Proof. Suppose V = Spec(f)(W) = f+1(W)
for a prime W of Y. Then f+1f-1(V)
is a subobject of X contained in V. But W 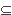 f-1(V) implies that V = f+1(W)
f-1(V) implies that V = f+1(W) 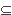 f+1f-1(V). Thus V = f+1f-1(V).
f+1f-1(V). Thus V = f+1f-1(V).
Conversely, assume V = f+1f-1(V)
holds. Consider the pullback (r: Z --> f-1(V),
s: Z --> P(V) of the maps (u: f-1(V)
--> V, p: P(V) --> V). Since u is epic and p is
coflat epic, s: Z --> p(V)
is epic, thus Z is non-initial, which has a simple subobject q:
Q --> Z. But psq = urq and the left side is epic, thus the right
side urq is epic. Let W = (rq)+1(Q).
Then u+1(W) = V. But u is the restriction
of f on f-1(V). This shows that f+1(W)
= V, i.e. V is lying in the image of Spec(f).

Proposition 5.2.3. Suppose f:
Y --> X is a coflat map.
(a} The image of f is {V Î
Spec(X): f-1(V) is non-initial}.
(b} If V is in the image of Spec(f) and W
is a integral strong subobject containing V, then W is in
the image (i.e. the image of Spec(f) is closed under generalizations).
Proof. (a) If V is in the image then by (5.2.2)
f+1f-1(V) = V, so f-1(V)
is non-initial.
Conversely, assume f-1(V) is non-initial.
The induced map t: f-1(V) --> V
is the pullback of f along V --> X, thus is also coflat.
Since V is integral and f-1(V) is non-initial,
t is epic by (3.2.6.a). Thus f+1f-1(V)
= V, which implies that V is in the image of Spec(f)
by (5.2.2).
 (b) If V Í W are two primes
of X and V is in the image of Spec(f), then
by (a) f-1(V) is non-initial. This implies that
f-1(W) is non-initial, so W is in the image
of Spec(f) by (a).
(b) If V Í W are two primes
of X and V is in the image of Spec(f), then
by (a) f-1(V) is non-initial. This implies that
f-1(W) is non-initial, so W is in the image
of Spec(f) by (a).
Proposition 5.2.4. Suppose Y
is a local object with the simple prime M and f: Y -->
X is a coflat mono. Then Spec(f) induces a homeomorphism
between Spec(Y) and the subspaces of Spec(X)
consisting of primes which contains M.
Proof. Since f is a coflat mono, Spec(f)
is a topological embedding by (3.6.9.a).
Since any prime V of Y contains M, we have f+1(V)  f+1(M)
f+1(M)  M. Conversely, if W is a prime of X which contains M,
then it contains f+1(M). Thus by (5.2.3.b)
it is in the image of Spec(f).
M. Conversely, if W is a prime of X which contains M,
then it contains f+1(M). Thus by (5.2.3.b)
it is in the image of Spec(f).
Proposition 5.2.5. Suppose f:
Y --> X is a coflat local map of local objects. Then Spec(f):
Spec(Y) --> Spec(X) is surjective.
Proof. By (3.4.7) the simple prime
P of X is in the image of f. Since any prime of X
contains P, by (5.2.3.b) it is in the
image of f.
Suppose V is an integral subobject of an object X. Denote
by XV the intersection of all the analytic subobjects
of X which is not disjoint with V.
Proposition 5.2.6. Assume V is
an integral subobject of X with the generic residue P(V).
(a) XV is the intersection of all the analytic subobjects
of X containing P(V).
(b) XV is a local object whose simple prime
contains P(V).
(c) XV is the intersection of all the fractions of
X containing P(V).
(d) If V is a prime then P(V) = XV  V.
V.
Proof. (a) Since V is integral and the simple object
P(V) is the intersection of non-initial fractions of V,
an analytic subobject of X is not disjoint with V iff it
contains P(V).
(b) XV contains P(V) by (a), thus is
non-initial. We prove that any non-initial strong subobject S of
XV contains P(V), which would implies that
the intersection P'(V) of all the non-initial strong subobjects
of XV is non-initial, and therefore XV
is local whose simple prime P'(V) contains P(V).
If this is not the case, then the simple object P(V) is disjoint
with S. Since XV is a coflat subobject of X,
S is induced by a strong subobject S' such that S
= S'  XV.
Then P(V) is disjoint with S'. Since A
is locally disjunctable, we can find a disjunctable strong subobject T
of X such that S' XV.
Then P(V) is disjoint with S'. Since A
is locally disjunctable, we can find a disjunctable strong subobject T
of X such that S' 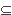 T and P(V) is not contained in T. Then the
simple object P(V) is disjoint with the strong subobject
T, so P(V)
T and P(V) is not contained in T. Then the
simple object P(V) is disjoint with the strong subobject
T, so P(V) 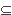 Tc, thus Tc is not disjoint with V,
so XV as the intersection of such analytic subobjects
is contained in Tc, which is absurd as S is in
XV but not in Tc as S
Tc, thus Tc is not disjoint with V,
so XV as the intersection of such analytic subobjects
is contained in Tc, which is absurd as S is in
XV but not in Tc as S 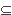 S'
S' 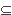 T. This shows that
any non-initial strong subobject S of XV contains
P(V), so XV is local. T. This shows that
any non-initial strong subobject S of XV contains
P(V), so XV is local.
(c) As P(V) ® XV
is local (thus quasi-local by (3.3.4.a)
and XV --> X is a faction, this pair of maps is
the quasi-local-fraction factorization of the inclusion P(V)
--> X. By (4.2.9) XV
is the intersection of fractions of X containing P(V).
(d) Clearly P(V) is contained in XV  V, thus XV
V, thus XV  V is non-initial, which is a fraction of V as XV
is a fraction of X. It suffices to prove that XV
V is non-initial, which is a fraction of V as XV
is a fraction of X. It suffices to prove that XV  V is simple. Consider a proper strong subobject S of XV
V is simple. Consider a proper strong subobject S of XV  V. Since XV
V. Since XV  V is a coflat subobject of V, S is induced by a proper
strong subobject S' of V, i.e. S = (XV
V is a coflat subobject of V, S is induced by a proper
strong subobject S' of V, i.e. S = (XV  V)
V)  S'. Since
V is a proper strong subobject of X, S' is also a
proper strong subobject of X. Since A
is locally disjunctable, we can find a disjunctable strong subobject T
of X containing S' but not V. Then T S'. Since
V is a proper strong subobject of X, S' is also a
proper strong subobject of X. Since A
is locally disjunctable, we can find a disjunctable strong subobject T
of X containing S' but not V. Then T  V is a proper subobject of V, and Tc
V is a proper subobject of V, and Tc  V = (T
V = (T  V)c.
Since V is reduced and T V)c.
Since V is reduced and T  V is proper, (T
V is proper, (T  V)cis a non-initial analytic subobject of V.
Since by definition XV
V)cis a non-initial analytic subobject of V.
Since by definition XV 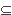 Tc
Tc  V
= (T V
= (T  V)c,
XV is disjoint with T V)c,
XV is disjoint with T  V. As S
V. As S 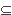 S' S' 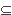 T
T  V, we see that XV
is disjoint with S. Since XV is non-initial
containing S, this means that S is initial. We have
proved that any proper strong subobject S of XV V, we see that XV
is disjoint with S. Since XV is non-initial
containing S, this means that S is initial. We have
proved that any proper strong subobject S of XV  V is non-initial. Thus XV
V is non-initial. Thus XV  V is simple.
V is simple.
Proposition 5.2.7. If P is a
simple subobject of X then XP is a local object,
and XP is the intersection of all the analytic
subobjects (or fractions) of X which contains P.
Proof. The assertion follows from (5.2.6).
Definition 5.2.8. (a) A fraction Y
--> X with a local domain
Y is called a localization.
(b) Suppose V is an integral subobject of an object X.
With the notations of (5.2.6), the localization lV:
XV --> X is called the localization
of X at V.
Remark 5.2.9. Suppose  is a small strong cogenerating set formed by finitely copresentable
objects. If W is a
is a small strong cogenerating set formed by finitely copresentable
objects. If W is a  -principal
regular subobject of an object X, we say that Wc
is a -principal
regular subobject of an object X, we say that Wc
is a  -principal
analytic subobject of X. Similar to (5.2.6)
and (5.2.7) one can show that for any integral subobject
V of X, the localization XV of
X at V is the intersection of -principal
analytic subobject of X. Similar to (5.2.6)
and (5.2.7) one can show that for any integral subobject
V of X, the localization XV of
X at V is the intersection of  -principal
analytic subobjects of X which is not disjoint with V (the
key step is (5.2.6.c) to prove that the intersection
is a local object). -principal
analytic subobjects of X which is not disjoint with V (the
key step is (5.2.6.c) to prove that the intersection
is a local object).
Proposition 5.2.10. Suppose f:
Y --> X is a localization and P is the simple prime
of the local object Y and V = f+1(P).
Then Y is the localization of X at the prime V.
Proof. Since f is coflat, P = f-1f+1(P)
= Y  f+1(P)
implies that P is a epic simple fraction, thus P is the generic
residue of the prime V = f+1(P). Since
the inclusion P --> Y is local and f: Y -->
X is a fraction, this pair of maps is the quasi-local-fraction factorization
of P --> X. But the inclusion P --> XV
is also local and the inclusion XV --> X
is a fraction, we obtain another pair of maps which is also a quasi-local-fraction
factorization of the inclusion P --> X. By the uniqueness
of such a factorization we see that Y = XV as
subobjects of X. f+1(P)
implies that P is a epic simple fraction, thus P is the generic
residue of the prime V = f+1(P). Since
the inclusion P --> Y is local and f: Y -->
X is a fraction, this pair of maps is the quasi-local-fraction factorization
of P --> X. But the inclusion P --> XV
is also local and the inclusion XV --> X
is a fraction, we obtain another pair of maps which is also a quasi-local-fraction
factorization of the inclusion P --> X. By the uniqueness
of such a factorization we see that Y = XV as
subobjects of X.
Proposition 5.2.11. Let f: Y
--> X be a map. Suppose W 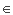 Spec(Y) and V = f+1(W). Then
there is a unique local map fW: YW
--> XV of local objects such that the following diagram
commutes:
Spec(Y) and V = f+1(W). Then
there is a unique local map fW: YW
--> XV of local objects such that the following diagram
commutes:
 Proof. Since XV is the intersection of analytic
subobjects of X which is not disjoint with V, f-1(XV)
contains YW, which is the intersection of analytic subobjects
of Y that is not disjoint with W. Thus the composition W
--> X of the inclusion W --> Y and f factors
through the inclusion XV --> X in a unique map
fW: YW --> XV. Clearly
f sends YW --> W = P(W) into
XV --> V = P(V), where P(W)
and P(V) are the generic residues of W and V
respectively. Thus fW is local by (3.4.7.b).
Proof. Since XV is the intersection of analytic
subobjects of X which is not disjoint with V, f-1(XV)
contains YW, which is the intersection of analytic subobjects
of Y that is not disjoint with W. Thus the composition W
--> X of the inclusion W --> Y and f factors
through the inclusion XV --> X in a unique map
fW: YW --> XV. Clearly
f sends YW --> W = P(W) into
XV --> V = P(V), where P(W)
and P(V) are the generic residues of W and V
respectively. Thus fW is local by (3.4.7.b).
With the notation of (5.2.11) we say that XV is the local
image of the localization YW. of Y (under
f).
Definition 5.2.12. Let f: Y
--> X be a map. We say that the going-up
theorem holds for f if the following condition
is satisfied:
For any V, V' 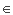 Spec(X) such that V
Spec(X) such that V 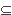 V', and for any W
V', and for any W 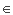 Spec(Y) with f+1(W) = V,
there exists W'
Spec(Y) with f+1(W) = V,
there exists W' 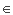 Spec(Y)
with f+1(W') = V' such that W Spec(Y)
with f+1(W') = V' such that W 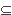 W'.
W'.
Now we can prove a generalization of the dual version of the going-down
theorem for a flat homomorphism of commutative rings (cf. [Matsumura
1980, p.33, Theorem 4]):
Proposition 5.2.13. (Going Up Theorem)
The going-up theorem holds for any coflat map f: Y --> X.
Proof. Suppose V, V' 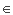 Spec(X) such that V
Spec(X) such that V 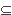 V', and W
V', and W 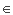 Spec(B)
with f+1(W) = V. Consider the localization
lW: YW --> Y, which is coflat. Then
flW: YW --> X is coflat. By (5.2.3.b)
V' is in the image of flW. Thus there is a prime
Q of YW such that flW+1(Q)
= V. Let W' = lW+1(Q).
Then W' contains W and f(W') = W as
desired (alternatively the theorem follows from the fact that the induced
local map YW --> XV is local, thus
the mapping Spec(YW) --> Spec(XV)
is surjective, and the fact that the mappings Spec(YW)
--> Spec(Y) and Spec(XV) --> X
are embeddings.). Spec(B)
with f+1(W) = V. Consider the localization
lW: YW --> Y, which is coflat. Then
flW: YW --> X is coflat. By (5.2.3.b)
V' is in the image of flW. Thus there is a prime
Q of YW such that flW+1(Q)
= V. Let W' = lW+1(Q).
Then W' contains W and f(W') = W as
desired (alternatively the theorem follows from the fact that the induced
local map YW --> XV is local, thus
the mapping Spec(YW) --> Spec(XV)
is surjective, and the fact that the mappings Spec(YW)
--> Spec(Y) and Spec(XV) --> X
are embeddings.).
[Next Section][Content][References][Notations][Home]
|



