Dave's Math Tables: Trigonometric Identities
(Math | Trig | Identities)

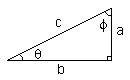
sin(theta) = a / c

csc(theta) = 1 / sin(theta) = c / a

cos(theta) = b / c

sec(theta) = 1 / cos(theta) = c / b

tan(theta) = sin(theta) / cos(theta) = a / b

cot(theta) = 1/ tan(theta) = b / a

sin(-x) = -sin(x)
csc(-x) = -csc(x)
cos(-x) = cos(x)
sec(-x) = sec(x)
tan(-x) = -tan(x)
cot(-x) = -cot(x)

sin^2(x) + cos^2(x) = 1

tan^2(x) + 1 = sec^2(x)

cot^2(x) + 1 = csc^2(x)

sin(x y) = sin x cos y cos x sin y

cos(x y) = cos x cosy sin x sin y

tan(x y) = (tan x tan y) / (1 tan x tan y)

sin(2x) = 2 sin x cos x

cos(2x) = cos^2(x) - sin^2(x) = 2 cos^2(x) - 1 = 1 - 2 sin^2(x)

tan(2x) = 2 tan(x) / (1 - tan^2(x))

sin^2(x) = 1/2 - 1/2 cos(2x)

cos^2(x) = 1/2 + 1/2 cos(2x)

sin x - sin y = 2 sin( (x - y)/2 ) cos( (x + y)/2 )

cos x - cos y = -2 sin( (x-y)/2 ) sin( (x + y)/2 )

Trig Table of Common Angles
angle 0 30 45 60 90
sin^2(a) 0/4 1/4 2/4 3/4 4/4
cos^2(a) 4/4 3/4 2/4 1/4 0/4
tan^2(a) 0/4 1/3 2/2 3/1 4/0

Given Triangle abc, with angles A,B,C; a is opposite to A, b oppositite B, c opposite C:

a/sin(A) = b/sin(B) = c/sin(C) (Law of Sines)

c^2 = a^2 + b^2 - 2ab cos(C)

b^2 = a^2 + c^2 - 2ac cos(B)

a^2 = b^2 + c^2 - 2bc cos(A)

(Law of Cosines)

(a - b)/(a + b) = tan 1/2(A-B) / tan 1/2(A+B) (Law of Tangents) --not neccessary with the above